“The only way to learn mathematics is to do mathematics.” - Paul R. Halmos”

Leveraging Base 10 Blocks for Multi-Sensory Math Tutoring 

If you are looking for a concrete way to help your student with dyscalculia understand basic number sense, perform operations, and improve mathematical reasoning skills, Base 10 Blocks are a terrific tool for providing multi-sensory support.

What are Base 10 Blocks?

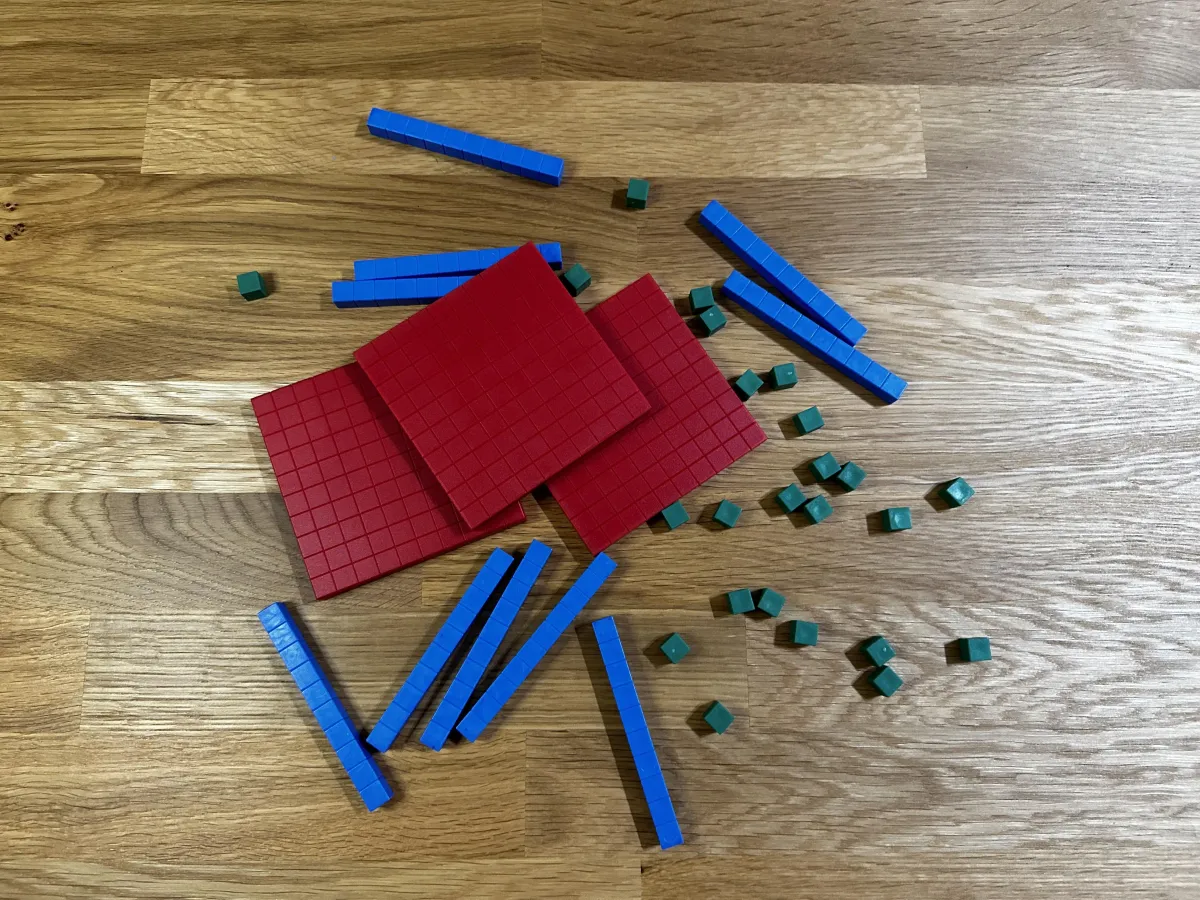
Base 10 Blocks are physical manipulatives that represent our number system, which is based on the number 10. These blocks provide a visual and tactile opportunity to engage with multi-digit numbers. Often, they are color-coded to help reinforce the differences in quantity.

Most often, you will find sets including ones or units (small green cubes), tens (blue rods equivalent to 10 units), hundreds (red flats equivalent to 100 units), and thousands (large green cubes equivalent to 1000 units). 

Students are able to manipulate these blocks and discover the relationship between units (ones), tens, hundreds, and thousands.

How do Base 10 Blocks help?

Students with dyscalculia learn math best when concepts are concrete and multi-sensory. Base 10 Blocks provides a concrete, colorful, hands-on learning opportunity where students can physically experience mathematical concepts.

By providing both a visual and tactile experience, using Base 10 Blocks helps students develop an understanding of place value, arithmetic, and our number system.

Students can start with the concrete representation of numbers using the blocks, then move to pictorial representations (drawing the blocks), and finally to abstract representations (writing numbers and equations).

This progression helps to bridge the gap between concrete and abstract thinking.

In what ways can Base 10 Blocks be used?

There are countless ways to use Base 10 Blocks to teach math. Here are a few examples:

Place Value: Students build numbers using the blocks (e.g. 1 flat, 3 rods, and 7 cubes is 137). To clearly illustrate the values of the ones, tens, and hundreds places, try having your student build a number where the digit repeats (e.g. 222). With two flats, 2 rods, and 2 unit cubes displayed, your student can visualize the quantity or value differences when a 2 is located in each of these places within the number 222. 

Comparing and Ordering Numbers: Have your student build two or more numbers and then either compare the values of each, or place them in order from least to greatest. These manipulatives give a concrete picture of the value of each number allowing the student to better see and understand the relationship between the numbers.

Addition: (with and without regrouping): To calculate 244+178, students create piles for each digit in each number and then combine them, beginning with the units and regrouping 10 of one kind for the next highest place value if needed. Trading between places becomes intuitive when they can physically exchange 10 ones for a ten, and so on.

Subtraction: (with and without regrouping): For 326-142, have your student build 326 and then remove 142, beginning with the units place and counting what's left.  The need to regroup before subtracting is noticeable for students when they cannot physically take away quantities without performing this critical step. If regrouping is required, the student should move the larger block to the column needing it before exchanging it for ten of the smaller blocks.

Multiplication: To multiply 14x12, make an array with 1 ten and 4 ones on one side, and 1 ten and 2 ones on the other. Students can count the total or break the array into smaller chunks (a hundreds flat, two tens rods, 4 tens rods and 8 ones cubes, totaling 168).

Division: For division, your student can distribute a large number of blocks into equal groups,  sharing equally beginning with the largest place value and exchanging for smaller blocks when needed for equal distribution.

Decimals: Base 10 Blocks are the perfect manipulative for teaching decimals because decimals are an extension of our base 10 number system. After your student has mastered place value of whole numbers, you can shift and redefine the value of your Base 10 Blocks to represent numbers less than one.  By assigning a value of 1.0 to the red flat, 0.1 to the blue rod, and .01 to the small green cube, you can teach all of the operations using decimals with these learning aids.  

Problem Solving with Critical Thinking: The blocks can be used to model and solve word problems, helping students to visualize the problem and develop problem-solving strategies. Encourage your students to use the blocks to work out solutions.

The Power of Multi-Sensory Learning

Working with Base 10 Blocks allows students with dyscalculia to build a concrete understanding of abstract math. The blocks make numbers tangible and operations visible. Students can manipulate something physical as they process the accompanying mathematical concepts. With time and practice, this multi-sensory learning helps struggling students build confidence and proficiency in math.

If you tutor a student with dyscalculia, consider incorporating Base 10 Blocks into your lessons. You'll be amazed at the difference this simple manipulative can make! With multi-sensory supports like Base 10 Blocks, all learners can experience success in mathematics.

To learn more about multi-sensory learning, visit my blog post "What is Multi-sensory Learning"